Factoring polynomials examples

If we completely factor a number into positive prime factors there will only be one way of doing it. That is the reason for factoring things in this way. You must be able to factor out of every term in order to identify the GCF.

We then divide by the corresponding factor to find the other factors of the expression. Factoring using roots. Similarly, in the case of polynomials, the factors are the polynomials which are multiplied to produce the original polynomial.

This method is used when you have a polynomial of degree greater than two, in order to simplify the expression to several polynomials of lesser degree. If all of the terms in a polynomial contain one or more identical factors, combine those similar factors into one monomial, called the greatest common factor, and rewrite the polynomial in factored form.

If you choose, you could then multiply these factors together, and you should get the original polynomial (this is a great way to check yourself on your factoring skills). Using Polynomials in Real Life.

Special products of Polynomials. Step-by-Step Examples. Algebra students learn about factoring polynomials by grouping.

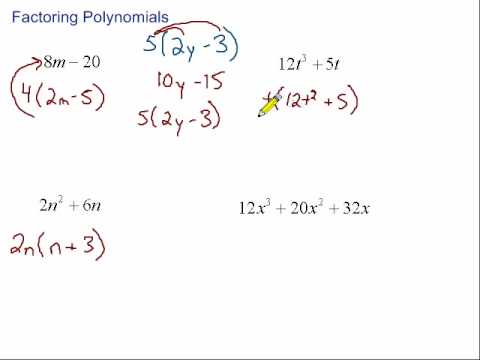
This video will explain how to factor a polynomial using the greatest common factor, trinomials and special factoring rules. Dividing Polynomials (Long Division) Dividing polynomials using long division is analogous to dividing numbers.

Similarly, we start dividing polynomials by seeing how many times one leading term fits into the other. The factoring of a polynomial refers to finding polynomials of lower order (highest exponent is lower) that, multiplied together, produce the polynomial being factored. Before you can factor trinomials, for example, you should check for any GCF.

In these lessons, we will look at factoring by common factors and factoring of polynomials by grouping. Scroll down the page for examples and solutions. Unsubscribe from Math Meeting?

Cool Math Guy 181views. Algebra factoring lessons with lots of worked examples and practice problems. Very easy to understand! Solution: The above polynomial can be factorized as.

Is not completely factored since the second factor can be factored some more. You can note that the first factor is completely factored. Let us look at the complete factorization of this polynomial. Many polynomial expressions can be written in simpler forms by factoring.

In this section, we will look at a variety of methods that can be used to factor polynomial expressions. When we studied fractions, we learned that the greatest common factor (GCF) of two numbers is the largest number that divides evenly into both numbers. In this case, we need to remove all parentheses by distributing and set the equation equal to zero with the terms written in descending order.

Note in these examples that we must always regard the entire expression. Polynomial Equation- is simply a polynomial that has been set equal to zero in an equation.